Answer:
This proves that f is continous at x=5.
Explanation:
Taking f(x) = 3x-1 and
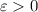 , we want to find a
, we want to find a
 such that
such that
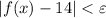
At first, we will assume that this delta exists and we will try to figure out its value.
Suppose that
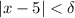 . Then
. Then
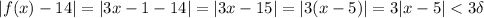 .
.
Then, if
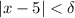 , then
, then
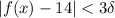 . So, in this case, if
. So, in this case, if
 we get that
we get that
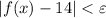 . The maximum value of delta is
. The maximum value of delta is
 .
.
By definition, this procedure proves that
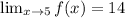 . Note that f(5)=14, so this proves that f is continous at x=5.
. Note that f(5)=14, so this proves that f is continous at x=5.