Question

In triangle ABC angle A=45 angle B =40 and a=7.Which equation should you solve to find b?

Answer:
Sin45 / 7 = sin40 / b

Answer 1

We have been given that in triangle ABC angle A=45 angle B =40 and a=7. We are asked to find the equation that we can use to solve for 'f'.

We have two angles and opposite sides to these angles.

We will use Law of Sines to solve our given problem.

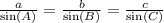`(a)/(\sin(A))=(b)/(\sin(B))=(c)/(\sin(C))`, where a, b and c are opposite sides corresponding to angles A, B, and C respectively.

The angle opposite to side b is 40 degrees and angle opposite to side a is 45 degrees.

Upon substituting these values in Law of Sines, we will get:

`(7)/(\sin(45^(\circ)))=(b)/(\sin(40^(\circ)))`

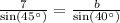
Therefore, our required equation would be
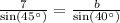`(7)/(\sin(45^(\circ)))=(b)/(\sin(40^(\circ)))`.