Question

Petrich is wrapping four presents for his mom, dad, sister, and daughter. The following are the estimate box sizes:

8.5" × 14.5" × 1.5"
9.5" × 14.5" × 2"
11" × 16" × 3"
15.5" × 12"× 4"

A standard roll of wrapping paper is 30" long with nine feet (three yards) of paper. Determine the minimum amount of rolls of wrapping paper that Petrich needs for all four presents.

Answer 1

Minimum 2 rolls will be required.

Explanation:

Surface area of all three boxes = Area of the wrapping paper required

Surface area of Box 1 = 2(lb + bh + hl)

= 2(8.5×14.5 + 14.5×1.5 + 8.5×1.5)

= 315.5 square inches

Surface area of box 2 = 2(9.5×14.5 + 14.5×2 + 9.5×2)

= 371.5 square inches

Surface area of box 3 = 2(15.5×12 + 12×4 + 15.5×4)

= 592 square inches

Surface area of box 4 = 2(11×16 + 16×3 + 11×3)

= 514 square inches

Total surface area = 315.5 + 371.5 + 592 + 514

= 1793 square inches

Dimensions of the wrapping paper = 30" × 9 feet Or 30" × 108"

Area of the wrapping paper = 3240 square inches

Number of papers required to wrap all four boxes =
`(3240)/(1793)=1.8`

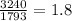
≈ 2 rolls

Therefore, minimum 2 rolls will be required to wrap the presents.