Question

Predict what the graph of the following quadratic function will look like. (If you have a graphing calculator, you can use it to verify your prediction.)

y = negative 3 x squared + 1

a.
Upward facing parabola-shaped; y-intercept (0, 1); symmetrical with respect to the y-axis
b.
Downward facing parabola-shaped; y-intercept (0, 1); symmetrical with respect to the y-axis
c.
Upward facing parabola-shaped; y-intercept (1, 0); assymmetrical with respect to the y-axis
d.
Downward facing parabola-shaped; y-intercept (1, 0); assymmetrical with respect to the y-axis

Answer 1

We have been given an equation
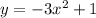`y=-3x^2+1`. We are asked to predict the graph of our given function.

We can see that our given equation is a quadratic function.

We know that vertex for of parabola is
`y=a(x-h)^2+k`, where a is leading coefficient and point (h,k) is vertex of parabola.

We can rewrite our given equation in vertex form as:

`y=-3(x-0)^2+1`

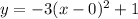
Upon comparing our given equation with vertex form, we can see that leading coefficient is
`-3` and vertex is at point
`(0,1)`.

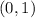Since vertex is at
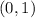`(0,1)`, so line of symmetry will be
`x=0` that is equation of y-axis. So parabola will be symmetric to y-axis.

Since leading coefficient is negative, therefore, our parabola will be downward opening and option 'b' is the correct choice.