Answer:
The thickness of the walls of each hollow lump of "iron ore" is 2.2 cm
Step-by-step explanation:
Here we have that the density of solid gold = 19.3 g/cm³
Density of real iron ore = 5.15 g/cm³
Diameter of sphere of gold = 4 cm
Therefore, volume of sphere = 4/3·π·r³ = 4/3×π×2³ = 33.5 cm³
Mass of equivalent iron = Density of iron × Volume of iron = 5.15 × 33.5
Mass of equivalent iron = 172.6 cm³
∴ Mass of gold per lump = Mass of equivalent iron = 172.6 cm³
Volume of gold per lump = Mass of gold per lump/(Density of the gold)
Volume of gold per lump = 172.6/19.3 = 8.94 cm³
Since the gold is formed into hollow spheres, we have;
Let the radius of the hollow sphere = a
Therefore;
Total volume of the hollow gold sphere = Volume of gold per lump - void sphere of radius, a
Therefore;
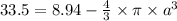
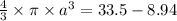
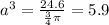
a = ∛5.9 = 1.8
The thickness of the walls of each hollow lump of "iron ore" = r - a = 4 - 1.8 = 2.2 cm.