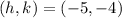Question

The process of completing the square is done for f(x) = x^2 +10x + 21 to be changed into a vertex form..which step shows Three steps toward this process? why did you pick your answer ?

Answer 1

And for this case we can begin completing the square like this:

`f(x) = x^2 +10x + (10/2)^2 +21 -(10/2)^2`

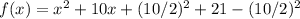
And after aggrupate the terms we got:

`f(x) = (x+5)^2 +21 -25`

And finally we have:

`f(X)= (x+5)^2 -4`

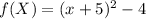
And for this case our vertex would be:

`(h,k) = (-5,-4)`

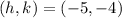
Explanation:

For this case we have the following equation given:

`f(x) = x^2 +10x +21`

And we want to find a formula in terms in the vertex form given by:

`f(x) = a(x-h)^2 +k`

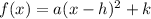
And for this case we can begin completing the square like this:

`f(x) = x^2 +10x + (10/2)^2 +21 -(10/2)^2`

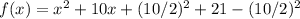
And after aggrupate the terms we got:

`f(x) = (x+5)^2 +21 -25`

And finally we have:

`f(X)= (x+5)^2 -4`

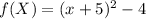
And for this case our vertex would be:

`(h,k) = (-5,-4)`