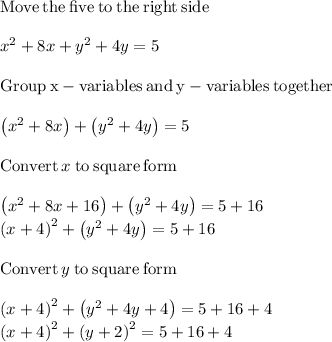
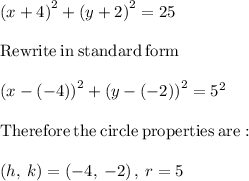Answer:
The standard form equation is
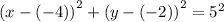 .
.
The center is
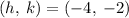 and the radius is
and the radius is
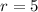 .
.
Explanation:
The standard form of an equation of a circle is
 where
where
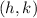 is the center and the radius is
is the center and the radius is
 .
.
To write the equation
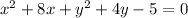 in the form of the standard circle equation you must:
in the form of the standard circle equation you must: