Answer:
BC = 9 miles
Explanation:
Here, we are given ΔABC ∼ ΔEDC, which means both triangles are similar and they tend to have the same dimensions and properties.
To find the length of BC, we are given the proportion:
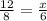
Where x represents the length BC.
Solving further, let's cross multiply
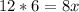
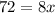
Solving for x, we have:
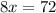
Lets divide both sides by 8
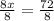
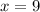
Therefore the distance BC is 9 miles