Question

What is the length of line segment KJ?

2 \sqrt{3} units

3 \sqrt{2} units

3 \sqrt{3} units

3 \sqrt{5} units

Answer 1

3√5

Explanation:

what is the length of line segment kJ

Answer 2

Please consider the attached file.

We can see that triangle JKM is a right triangle, with right angle at M. Segment KM is 6 units and segment MJ is 3 units. We can also see that KJ is hypotenuse of right triangle.

We will use Pythagoras theorem to solve for KJ as:

`KJ^2=KM^2+MJ^2`

`KJ^2=6^2+3^2`

`KJ^2=36+9`

`KJ^2=45`

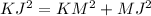
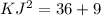
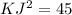
Now we will take positive square root on both sides:

`√(KJ^2)=√(45)`

`KJ=√(9\cdot 5)`

`KJ=3√(5)`

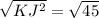
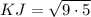
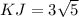
Therefore, the length of line segment KJ is
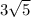`3√(5)` and option D is the correct choice.