Question

What is the true solution to the equation below?

2 In elin2x - In en 10x – In 30

O x-30

O x-75

O x-150

O x=300

Answer 1

Explanation:

some rules of logarithmic function

`ln(a) - ln(b)=ln((a)/(b))`

`e^(ln(a))=a`

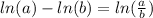
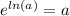
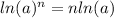`ln(a)^(n)=nln(a)` vice-versa
`nln(a)=ln(a)^(n)`

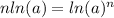
If ㏑(a) = ㏑(b), then a = b

∴
`2ln(e^(ln(2x)))-ln(e^(ln(10x)))=ln(30)`

Use the 2nd rule to simplify it

`e^(ln(2x))=2x\\e^(ln(10x))=10x\\`

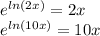
2㏑(2x) - ㏑(10x) = ㏑(30)

Use the 3rd rule in the 1st term

∵ 2㏑(2x) = ㏑(2x)² = ㏑(4x²)

∴ ㏑(4x²) - ㏑(10x) = ㏑(30)

- Use the 1st rule with the left hand side

`ln(4x^(2))-ln(10x)=ln((4x^(2))/(10x))\\\\ln((4x^(2))/(10x))=ln(30)\\\\ (4x^(2))/(10x)=(2x)/(5)=(2)/(5)x\\\\ ln((2)/(5)x)=ln(30)`

Use the 4th rule

`(2)/(5) x = 30`

Multiply both sides by 5

∴ 2 x = 150

- Divide both sides by 2

∴ x = 75

The value of x = 75