Question

The standard configuration for an Arizona license plate is 3 digits (0 - 9) followed by 3 letters (of 26). If you can not repeat digits or letters, how many plates (NO COMMAS NEEDED) can be made?

Answer 1

The number of plates that can be made without repetition is 3124550.

Explanation:

The appropriate way to determine the number of plates to be made is by the application of combination.

Since the digits has 9 elements and the letters have 26, then 26
`C_(9)`.

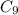So that:

n
`C_(r)` =
`(n!)/((n - r)!r!)`

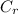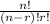
n = 26 and r = 9;

26
`C_(9)` =
`(26!)/((26 - 9)9!)`

=
`(26!)/(17!9!)`

=
`(26*25*24*23*22*21*20*19*18*17!)/(9*8*7*6*5*4*3*2*1*17!)`

=
`(1.133836704*10^(12) )/(362880)`

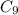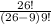
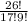
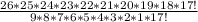
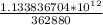
= 3124550

The number of plates that can be made without repeating numbers and letters is 3124550.