Question

HELP I HAVE NO IDEA WHATS GOING ON PLS HELP

This season, the probability that the Yankees will win a game is 0.49 and the probability that the Yankees will score 5 or more runs in a game is 0.56. The probability that the Yankees lose and score fewer than 5 runs is 0.36. What is the probability that the Yankees will win when they score fewer than 5 runs? Round your answer to the nearest thousandth.

Answer 1

0.182 probability that the Yankees will win when they score fewer than 5 runs

Explanation:

We use the conditional probability formula to solve this question. It is

`P(B|A) = (P(A \cap B))/(P(A))`

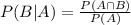
In which

P(B|A) is the probability of event B happening, given that A happened.

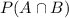`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

In this problem:

When the Yankees score less than 5 runs, either they win, or they lose. The sum of these probabilities is 1.

Probability they lose:

Event A: Scoring fewer than 5 runs.

Event B: Losing

The probability that the Yankees will score 5 or more runs in a game is 0.56.

So 1 - 0.56 = 0.44 probability the Yankees score fewer than 5 runs.

This means that
`P(A) = 0.44`

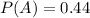
The probability that the Yankees lose and score fewer than 5 runs is 0.36.

This means that
`P(A \cap B) = 0.36`

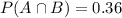
Then the probability they lose is:

`P(B|A) = (P(A \cap B))/(P(A)) = (0.36)/(0.44) = 0.818`

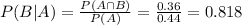
Probability they win:

p + 0.818 = 1

p = 0.182

0.182 probability that the Yankees will win when they score fewer than 5 runs