Question

A circle with radius 5 has a sector with a central angle of 9/10 pi

radians.

What is the area of the sector?

Either enter an exact answer in terms of it or use 3.14 for and enter your answer as a decimal rounded to the

nearest hundredth.

Answer 1

The area of the sector to nearest hundredth is 35.33

Explanation:

Formula for calculating the area of a sector is given as
`(\theta)/(360) *\pi r^(2)` where;

r is the radius of the circle

theta is the angle substended by the sector.

Given r = 5 and central angle theta =
`(9 \pi)/(10)`

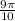
Area of the sector is expressed as shown;

`= (9\pi/10)/(2\pi)*\pi (5)^(2) \\= (9\pi)/(20\pi)*25\pi\\ = (225\pi)/(20) \\= 225*3.14/20\\= 35.33`

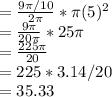
The area of the sector to nearest hundredth is 35.33

Answer 2

35.33

Explanation:

The circle with radius 5 has a sector with a central angle of 9/10 pi.

The area of a sector is given as:

`A_s = (\alpha )/(2\pi) * \pi r^2`

where α = central angle of the sector in radians

r = radius of the circle

The area of the sector is therefore:

`A_s = ((9 \pi)/(10) )/(2 \pi) * (3.14 * 5^2)\\ \\A_s = (9)/(20) * 78.5\\\\A_s = 35.33`

The area of the sector is 35.33.