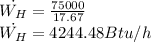Question

A heat pump is to be used for heating a house in winter. The house is to be maintained at 70°F at all times. When the temperature outdoors drops to 40°F, the heat losses from the house are estimated to be 75,000 Btu/h. Determine the minimum power [Btu/h] required to run this heat pump if heat is extracted from the outdoor air at 40°F.

Answer 1

`\dot{W_(H) } = 4244.48 Btu/h`

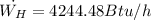
Step-by-step explanation:

Temperature of the house,
`T_(H) = 70^(0) F`

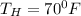
Convert to rankine,
`T_(H) = 70^(0)+ 460 = 530 R`

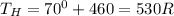
Heat is extracted at 40°F i.e
`T_(L) = 40^(0)F = 40 + 460 = 500 R`

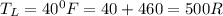
Calculate the coefficient of performance of the heat pump, COP

`COP = (T_(H) )/(T_(H) - T_(L) ) \\COP = (530 )/(530 - 500 )\\ COP = (530)/(30) \\COP = 17.67`

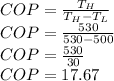
The minimum power required to run the heat pump is given by the formula:

`\dot{W_(H) } = \frac{\dot{Q_(H) }}{COP} \\`...............(*)

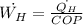Where the heat losses from the house,
`\dot{Q_(H) } = 75,000 Btu/h`

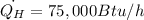
Substituting these values into * above

`\dot{W_(H) } = (75000)/(17.67) \\ \dot{W_(H) } = 4244.48 Btu/h`