Question

The manufacturer of hardness testing equipment uses​ steel-ball indenters to penetrate metal that is being tested.​ However, the manufacturer thinks it would be better to use a diamond indenter so that all types of metal can be tested. Because of differences between the two types of​ indenters, it is suspected that the two methods will produce different hardness readings. The metal specimens to be tested are large enough so that two indentions can be made.​ Therefore, the manufacturer uses both indenters on each specimen and compares the hardness readings. Construct a​ 95% confidence interval to judge whether the two indenters result in different measurements.

Answer 1

Check the explanation

Explanation:

Let X denotes steel ball and Y denotes diamond

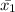`\bar{x_1}` = 1/9( 50+57+......+51+53)

=530/9

=58.89

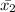`\bar{x_2}`= 1/9( 52+ 56+....+ 51+ 56)

=543/9

=60.33

difference = d =(60.33- 58.89)

=1.44

`s^2=1/n\sum xi^2 - n/(n-1)\bar{x}^2`

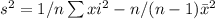
s12 = 1/9( 502+572+......+512+532) -9/8 (58.89)2

=31686/8 - 9/8( 3468.03)

=3960.75 - 3901.53

=59.22

s1 = 7.69

s22 = 1/9( 522+ 562+....+ 512+ 562) -9/8 (60.33)2

=33295/8 - 9/8 (3640.11)

=4161.875 - 4095.12

=66.75

s2 =8.17

sample standard deviation for difference is

s=
`√([(n1-1)s_1^2+ (n2-1)s_2^2]/(n1+n2-2))`

=
`√([(9-1)*59.22+ (9-1)*66.75]/(9+9-2))`

=
`√(1007.76/16)`

=7.93

sd =
`s*√((1/n1)+(1/n2))`

=
`7.93*√((1/9)+(1/9))`

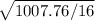
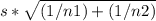
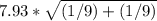
=7.93* 0.47

=3.74

For 95% confidence level
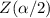`Z (\alpha /2)` =1.96

confidence interval is

`d\pm Z(\alpha /2)*s_d`

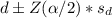
=(1.44 - 1.96* 3.75 , 1.44+1.96* 3.75)

=(1.44 - 7.35 , 1.44 + 7.35)

=(-2.31, 8.79)

There is sufficient evidence to conclude that the two indenters produce different hardness readings.