Answer:
The test statistic Z = 1.844 < 1.96 at 0.05 level of significance
Null hypothesis is accepted
Yes he is right
The manager claims that at least 95 % probability that the plant is operating properly
Explanation:
Explanation:-
Given data Population mean
μ = 885 tons /day
Given random sample size
n = 60
mean of the sample
x⁻ = 875 tons/day
The standard deviation of the Population
σ = 42 tons/day
Null hypothesis:- H₀: The manager claims that at least 95 % probability that the plant is operating properly
Alternative Hypothesis :H₁: The manager do not claims that at least 95 % probability that the plant is operating properly
Level of significance = 0.05
The test statistic

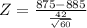
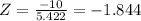
|Z| = |-1.844| = 1.844
The tabulated value

The calculated value Z = 1.844 < 1.96 at 0.05 level of significance
Null hypothesis is accepted
Conclusion:-
The manager claims that at least 95 % probability that the plant is operating properly