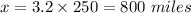Answer:800 miles
Explanation:
Given
Round trip for Penthaven to Jackson takes 6 hr and 24 minutes in absence of wind
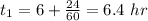
When Wind blows from Penthaven to Jackson it takes 6 hr and 40 min i.e.
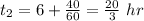
Speed of wind
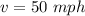
Suppose x be the distance between Penthaven and Jackson and u be the speed of plane
So initially
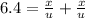
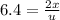
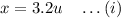
When wind is blowing then,
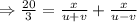
![\Rightarrow (20)/(3)=x[(1)/(u+50)+(1)/(u-50)]](https://img.qammunity.org/2021/formulas/mathematics/college/wvp5q0poq60y8f0gjsqnu175wq6aia5my1.png)
![\Rightarrow (20)/(3)=x[(2u)/(u^2-50^2)]\quad \ldots(ii)](https://img.qammunity.org/2021/formulas/mathematics/college/inp9hma7mrpj0p9pfzhlumh1r6zd26pl60.png)
Substitute the value of x in
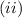
![\Rightarrow (20)/(3)=(2u[3.2u])/(u^2-50^2)](https://img.qammunity.org/2021/formulas/mathematics/college/7l3yfh70qybgbp2cf3dy2p0h7td20zkqi9.png)
![\Rightarrow 10[u^2-50^2]=9.6u^2](https://img.qammunity.org/2021/formulas/mathematics/college/7aie0567ddl2lkihrwv219khl7t6s2im9m.png)
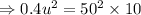
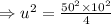
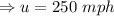
Thus