Answer:
It cost $0.91 10 years ago.
It takes 10.24 years for the cost of bread to double.
Explanation:
The equation for the price of bread after t years has the following format:
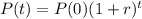
In which P(0) is the current price, and r is the inflation rate, as a decimal.
If we want to find the price for example, 10 years ago, we find P(-10).
Inflation is at a rate of 7% per year. Evan's favorite bread now costs $1.79.
This means that
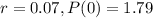 . So
. So
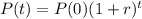
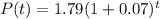

What did it cost 10 years ago?
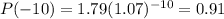
It cost $0.91 10 years ago.
How long before the cost of the bread doubles?
This is t for which P(t) = 2P(0) = 2*1.79. So

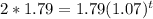
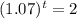
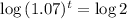
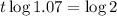

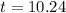
It takes 10.24 years for the cost of bread to double.