Question

A university found that 20% of its students withdraw without completing the introductory statistics course. Assume that 20 students registered for the course. (Round your answers to four decimal places.) (a) Compute the probability that 2 or fewer will withdraw. (b) Compute the probability that exactly 4 will withdraw. (c) Compute the probability that more than 3 will withdraw. (d) Compute the expected number of withdrawals.

Answer 1

(a) Probability that 2 or fewer will withdraw is 0.2061.

(b) Probability that exactly 4 will withdraw is 0.2182.

(c) Probability that more than 3 will withdraw is 0.5886.

(d) The expected number of withdrawals is 4.

Explanation:

We are given that a university found that 20% of its students withdraw without completing the introductory statistics course.

Assume that 20 students registered for the course.

The above situation can be represented through binomial distribution;

`P(X =r) = \binom{n}{r} * p^(r) * (1-p)^(n-r);x=0,1,2,3,......`

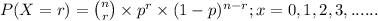
where, n = number of trials (samples) taken = 20 students

r = number of success

p = probability of success which in our question is probability

that students withdraw without completing the introductory

statistics course, i.e; p = 20%

Let X = Number of students withdraw without completing the introductory statistics course

So, X ~ Binom(n = 20 , p = 0.20)

(a) Probability that 2 or fewer will withdraw is given by = P(X
`\leq` 2)

P(X
`\leq` 2) = P(X = 0) + P(X = 1) + P(X = 2)

=
`\binom{20}{0} * 0.20^(0) * (1-0.20)^(20-0)+ \binom{20}{1} * 0.20^(1) * (1-0.20)^(20-1)+ \binom{20}{2} * 0.20^(2) * (1-0.20)^(20-2)`

=
`1 *1 * 0.80^(20)+ 20 * 0.20^(1) * 0.80^(19)+ 190* 0.20^(2) * 0.80^(18)`

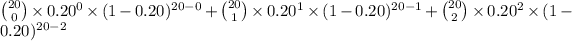
= 0.2061

(b) Probability that exactly 4 will withdraw is given by = P(X = 4)

P(X = 4) =
`\binom{20}{4} * 0.20^(4) * (1-0.20)^(20-4)`

=
`4845* 0.20^(4) * 0.80^(16)`

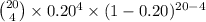
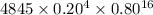
= 0.2182

(c) Probability that more than 3 will withdraw is given by = P(X > 3)

P(X > 3) = 1 - P(X
`\leq` 3) = 1 - P(X = 0) - P(X = 1) - P(X = 2) - P(X = 3)

=
`1-(\binom{20}{0} * 0.20^(0) * (1-0.20)^(20-0)+ \binom{20}{1} * 0.20^(1) * (1-0.20)^(20-1)+ \binom{20}{2} * 0.20^(2) * (1-0.20)^(20-2)+\binom{20}{3} * 0.20^(3) * (1-0.20)^(20-3))`

=
`1-(1 *1 * 0.80^(20)+ 20 * 0.20^(1) * 0.80^(19)+ 190* 0.20^(2) * 0.80^(18)+1140* 0.20^(3) * 0.80^(17))`

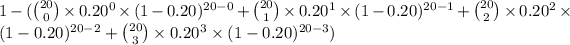
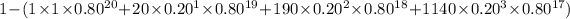
= 1 - 0.4114 = 0.5886

(d) The expected number of withdrawals is given by;

E(X) =
`n* p`

=
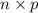
`20 * 0.20` = 4 withdrawals