Question

In ΔHIJ, the measure of ∠J=90°, the measure of ∠H=84°, and HI = 69 feet. Find the length of IJ to the nearest tenth of a foot.

Answer 1

68.622 ~ (68.6)feet

Explanation:

Answer 2

The length of IJ is 68.7 feet.

Explanation:

According to trigonometric identities for a right angled triangle, the sin of an angle is:

`sin\ \theta=\frac{\text{Perpendicular}}{\text{Hypotenuse}}`

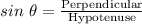
From the provided data we can interpret that:

Perpendicular = x feet

Hypotenuse = 69 feet

θ = 84°

Compute the value of x as follows:

`sin\ \theta=\frac{\text{Perpendicular}}{\text{Hypotenuse}}`

`sin\ 84^{\text{o}}=(x)/(69)\\\\0.995=(x)/(69)\\\\x=69*0.995\\\\x=68.655\\\\x\approx 68.7`

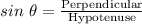
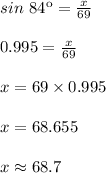
Thus, the length of IJ is 68.7 feet.