Answer:
a) The null and alternative hypotesis are:
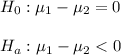
1: productivity when boss absent, 2: productivity when boss present
b) t=-3.492
c) df=60
d) tc=-1.6706
e) Yes, it is significant.
Explanation:
Hypothesis test for the difference between means.
The claim is that productivity decreases when the boss leaves the office.
Then, the null and alternative hypotesis are:
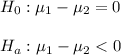
The significance level is α=0.05.
The degrees of freedom are calculated as:

The difference between sample means Md is:
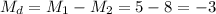
Now, we have to calculate the standard error for the difference of the means.
As the sample sizes are not equal, we have to calculate the harmonic mean of the sample size:
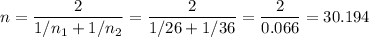
The standard deviation of sample 1 (boss absent) is:
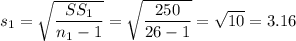
The standard deviation of sample 2 (boss present) is:
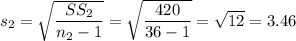
We calculate the mean square error (MSE) as:
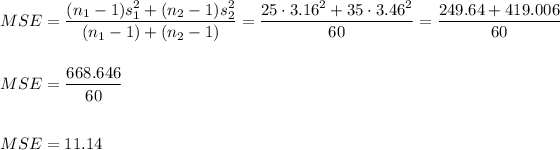
Then, the standard error can be calculated as:

Now, we can calculate the test statistic t:
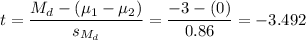
If we apply the critical value approach, the critical value of t for a significance level α=0.05 and 60 degrees of freedom is:
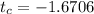
As this is a left-tail test, the decision rule is to reject the null hypothesis if the test statistic is smaller than the critical value.
In this case, the test statistic t=-3.492 is smaller than the critical value t_c=-1.6706, the effect is significant.