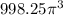Question

A circle with radius 33π has a sector with a central angle of 11/6π radians . What is the area of the sector?

Answer 1

Explanation:

Answer 2

the area of the sector with a central angle of 11/6π radians is equal to
`998.25\pi^3`

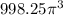
Explanation:

The area A of the entire circle is given by:

`A=\pi *r^(2)`

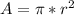
Where r is the radius of the circle. So the area of a circle with radius
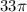`33\pi` is:

`A=\pi *(33\pi)^2=1089\pi^3`

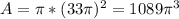
Additionally an entire circle has a central angle of
`2\pi` radians.

So, we can calculate the area of a sector using the rule of three in which we know that
`2\pi` radians has an Area of
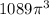`1089\pi^3` then what is the area of the sector with a central angle of 11/6π radians as:

`1089\pi^3 ------------2\pi\\ x--------------(11)/(6)\pi`

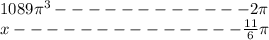
Where x is the area of the sector with a central angle of 11/6π radians.

Finally, solving for x, we get:

`x=(11/6\pi *1089\pi^3 )/(2\pi ) =998.25\pi^3`

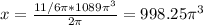
So, the area of the sector with a central angle of 11/6π radians is equal to
`998.25\pi^3`