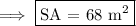Part I: Renaming the faces of the prism
Let the vertices of the prism be A, B, C, D, E, F, G, and H. (Refer to image).
From the image, we can tell that the faces of the prism are:
ABFG HDCE FBCE GADH GFEH ABCD
Part II: Recalling the surface area formula
Surface area formula:
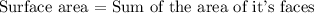
The faces are ABFG, HDCE, FBCE, GADH, GFEH, and ABCD (Previous Part)
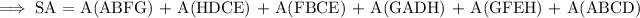
Part III: Determining the surface area of the prism
If we look at the prism carefully, we can tell that:
ABFG = DCEH
GADH = FBCE
GFEH = ABCD
Therefore, the new equation is:
![\implies \text{SA = 2[A(ABFG)] + 2[A(GADH)] + 2[A(GFEH)]}](https://img.qammunity.org/2023/formulas/mathematics/high-school/t9suum0c507p4q0ykvaj9osjgusgmk319g.png)
If we look carefully, we can see that GADH and ABFG are rectangles. Therefore, their areas will be length multiplied by width. Now, substitute the dimensions of the rectangles in the surface area.
![\implies \text{SA} = 2[4 * 4] + 2[2.5 * 4] + \text{2[A(GFEH)]}](https://img.qammunity.org/2023/formulas/mathematics/high-school/ory1pxgp5adcngy4yl4j2h5seh7autzm7c.png)
The area of a parallelogram is the base multiplied by the height.
![\implies \text{SA}= 2[4 * 4] + 2[2.5 *4] + 2[4 * 2]}](https://img.qammunity.org/2023/formulas/mathematics/high-school/t40cxeplun10fxzfbtelil90wa9ewgki6m.png)
Finally, simplify the equation to determine the surface area.
![\implies \text{SA} = 2[16] + 2[10] + 2[8]](https://img.qammunity.org/2023/formulas/mathematics/high-school/ddcs09a2fa7jx60lzofyj024ody15zfg0c.png)