Answer:
Correct option: Subtract Two-fifths from both sides.
Explanation:
The inequality is:
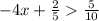
The best first step to solve the inequality will be to collect the like term on one side.
To do this, we must subtract
 from both sides of the inequality.
from both sides of the inequality.
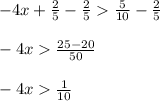
Now solve for x as follows:
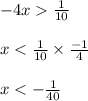
Thus, the value of x lies in the range
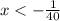 .
.