Question

The living spaces of all homes in a city have a mean of square feet and a standard deviation of square feet. Let be the mean living space for a random sample of homes selected from this city. Find the mean of the sampling distribution of . Enter an exact answer. mean of square feet Find the standard deviation of the sampling distribution of . Round your answer to one decimal place. standard deviation of square feet

Answer 1

`\bar X \sim N(\mu, (\sigma)/(√(n)))`

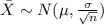
The mean for this case is given by:

`\mu_(\bar X) = 2300.0`

And the deviation would be:

`\sigma_(\bar X)= (500)/(√(25))= 100.0`

Explanation:

Using the following problem info since is incomplete the data: "The living spaces of all homes in a city have a mean of 2300 square feet and a standard deviation of 500 square feet. Let x be the mean living space for a random sample of 25 homes selected from this city. Find the mean and standard deviation of the sampling distribution of x."

We know the following info given:

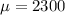`\mu = 2300` represent the mean for tehe living spaces of all homes in a city

`\sigma = 500` represent the population deviation for the data

We select a sample size of n=25 so then this size is large enough in order to use the central limit theorem and we can use for the distribution of the sampel mean:

`\bar X \sim N(\mu, (\sigma)/(√(n)))`

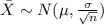
The mean for this case is given by:

`\mu_(\bar X) = 2300.0`

And the deviation would be:

`\sigma_(\bar X)= (500)/(√(25))= 100.0`