Question

A sample of 84 full electric cars was randomly drawn from a population with an unknown mean range μ (miles per charge) and a standard deviation σ of 25 (miles per charge). The cars in the sample have an average range of 125 (miles per charge). (a)[9] At the level of significance α = 0.04, test H0: μ ≥ 130 versus H1: μ < 130. Sketch the test. (b)[5] Sketch & find the p‐value. Would you accept the null if: α = 0.01; α = 0.05; and α = 0.10.

Answer 1

(a) The test statistic value is -1.83.

(b) We accept the null hypothesis at α = 0.01.

Explanation:

The information provided is:

`n=84\\\bar x=125\\\sigma=25\\\alpha =0.04`

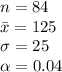
The hypothesis is defined as follows:

H₀: μ ≥ 130 versus Hₐ: μ < 130.

Since the population standard deviation is provided, we will use a z-test.

Compute the test statistic as follows:

`z=(\bar x-\mu)/(\sigma/√(n))=(125-130)/(25/√(84))=-1.83`

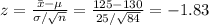
The test statistic value is -1.83.

(b)

Compute the p-value of the test as follows:

p-value = P (Z < -1.83)

= 0.034

* Use a z-table.

We reject a hypothesis if the p-value of a statistic is lower than the level of significance α.

• p-value = 0.034 < α = 0.04. Reject the null hypothesis.

• p-value = 0.034 > α = 0.01. Fail to reject the null hypothesis.

• p-value = 0.034 < α = 0.05. Reject the null hypothesis.

• p-value = 0.034 < α = 0.10. Reject the null hypothesis.