Answer:
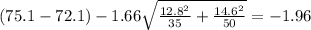
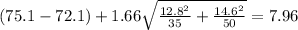
And the confidence interval would be given by:
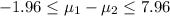
And since the confidence interval contains the value 0 we have enough evidence to conclude that we don't have significant differences between the two means at 10% of significance.
Explanation:
For this case we have the following info given :
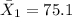 represent the sample mean for the scores of the undergraduate students
represent the sample mean for the scores of the undergraduate students
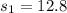 represent the standard deviation for the undergraduate students
represent the standard deviation for the undergraduate students
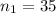 the sample size for the undergraduate
the sample size for the undergraduate
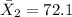 represent the sample mean for the scores of the high school students
represent the sample mean for the scores of the high school students
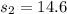 represent the standard deviation for the high school students
represent the standard deviation for the high school students
 the sample size for the high school
the sample size for the high school
The confidence interval for the true difference of means is given by:
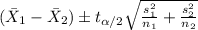
The degrees of freedom are given by:

The confidence level is 90% and the significance level is
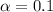 and
and
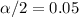 then the critical value would be:
then the critical value would be:
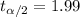
And replacing the info we got:
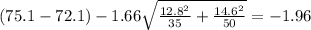
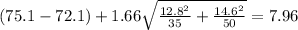
And the confidence interval would be given by:
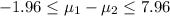
And since the confidence interval contains the value 0 we have enough evidence to conclude that we don't have significant differences between the two means at 10% of significance.