Question

A 15 mm × 15 mm silicon chip is mounted such that the edges are flush in a substrate. The chip dissipates 1.4 W of power uniformly, while air at 20°C (1 atm) with a velocity of 23.4 m/s is used to cool the upper surface of the chip. If the substrate provides an unheated starting length of 15 mm, determine the surface temperature at the trailing edge of the chip. Evaluate the air properties at 50°C. The properties of air at 50°C are k = 0.02735 W/m∙K, ν = 1.798 × 10−5 m2/s, and Pr = 0.7228.

Answer 1

107.1° C

Step-by-step explanation:

The properties of air from table '' properties of air at 1 atm pressure'' recorded at temperature T = 50°C

Thermal conductivity k = 0.02735 W/m.K

Kinematic viscosity v = 1.798 × 10⁻⁵ m²/s

Prandtl number Pr = 0.7228

Determine the characteristics length , L

`L = x + E`

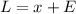
where;

x represent the length of the chip

E represent the unheated stating length

Replacing 15 mm for x and 15 mm for E

L = 15 + 15

L = 30 mm

To calculate the Reynolds number for the flow at the trailing edge (x = L + E)

`Re = (VL)/(v)`

Here;
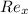`Re_x` is the Reynolds number and L is the characteristics length

Replacing 23.4 m/s for V , 30 mm for L and 1.798 × 10⁻⁵ m²/s for v

Re =
`(23.4((15*10^(-3))+(15*10^(-3)))/(1.798*10^(-5))`

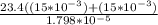
Re = 20355.95106

Thus, since the obtained Reynolds number is less than
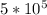`5*10^5` i.e Re <

To Calculate the local Nusselt number
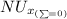`NU_{x_(( \sum = 0) )}` for the laminar flow with uniform heat flux ; we have :

`NU_{x_(( \sum = 0) )} = 0.453 Re^(0.5)Pr^(1/3)`

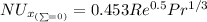
Replacing 20355.95106 for Re and 0.7228 for Pr; we get:

`NU_{x_(( \sum = 0) )} = 0.453(20355.95106) ^(0.5)(0.7228)^(1/3)`

`NU_{x_(( \sum = 0) )} =58.0`

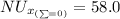
Calculate the Nusselt number for the silicon chip whose edges are flush in substrate

`Nu_x = \frac{NU_{x_(( \sum = 0) )}}{[1-( E/L)^(3/4)]^(1/3)}`

Replacing
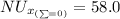`NU_{x_(( \sum = 0) )} =58.0` , 30 mm for L and 15 mm for E

`Nu_x = (58.0)/([1-( 15*10^(-3)/0.03)^(3/4)]^(1/3))`

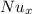`Nu_x` = 78.37

To the determination of the local heat coefficient

`Nu_x = (h_xL)/(k)`

making
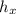`h_x` the subject of the formula

`h_x = (Nu_x*k)/(L)`

`h_x = (78.37*0.02735)/(0.03)`

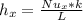
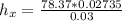
= 71.44 W/m² . K

Considering Newton's Law of cooling and calculating for the surface temperature; we have:

`Q = h_xA_s (T_s - T \infty) \\ \\ Q = h_x *x^2 (T_s - T \infty)`

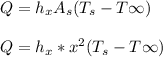
making
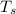`T_s` the subject of the formula

`T-s = T_ \infty + (Q)/(h_xx^2)`

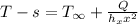
where Q = heat transfer = 1.4 W

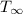`T_ \infty` = ambient temperature 20° C

`T_s = 20 + (1.4)/(71.44*(15*10^(-3))^2)`

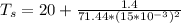
= 107.1° C

Thus, the surface temperature at the trailing edge of the chip is = 107.1° C