Question

If mJI = (3x+2)°, mHLK = (15x-36)°, and m∠HML = (8x-1)°, find mHLK

Answer 1

204

Explanation:

just did it

Answer 2

The value of mHLK will be "(204)°".

Explanation:

The given values are:

mJI = (3x+2)°

mHLK = (15x-36)°

and,

m∠HML = (8x-1)°

then,

mHLK = ?

Now,

By using chord-chord formula of angle, we get

`mHMK=(1)/(2)(mJL+mHLK)`

On putting the values in the above formula, we get

⇒
`(8x-1)=(1)/(2)(15x-36+3x+2)`

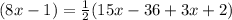
On applying cross-multiplication, we get

⇒
`2(8x-1)=18x-34`

⇒
`16x-2=18x-34`

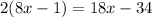
On subtracting "18x" from both sides, we get

⇒
`16x-2-18x=18x-34-18x`

⇒
`-2x-2=-34`

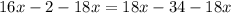
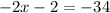
On adding "2" both sides, we get

⇒
`-2x=-34+2`

⇒
`-2x=-32`

⇒
`x=(32)/(2)`

⇒
`x=16`

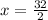
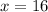
On putting the value of "x" in mHLK = (15x-36)°, we get

⇒ (15x-36)° = (15×16-36)°

⇒ = (240-36)°

⇒ = (204)°

So that mHLK = (204)°