Question

Find the area of the shaded region

Answer 1

20.52cm^2

Explanation:

from the picutre we can see that this is a semicircle with 2 right angle triangles inside it. to find the area we will solve for the semi circle then solve for the triangles and and subtract

circle area is
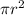`\pi r^(2)` leaving the semicircle to be
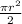`(\pi r^(2) )/(2)` for the semi circle

next we need the radius. since the diameter is given, we can solve for r by dividing it in two

12 ÷ 2 = 6

so the area of the semi circle is
`(\pi 6^(2) )/(2)`

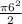
this gives 18
`\pi`cm^2 if we simplify

next the triangles, since they are equal in size we can treat them as a square and stack them. this is because they have equal side lengths so when stacked they form a square:

base x height divided by 2 times 2 would be the normal process to find two equal triangles but you can see the division and multiplication cancel out

6x6= 36cm^2

finally subtracting the areas to find the shaded region and use 3.14 as pi

18
`\pi`cm^2 - 36cm^2 = 20.52cm^2

Answer 2

20.52 cm²

Explanation:

`\textsf{Area of a semicircle}=\sf \frac12\pi r^2 \quad \textsf{(where r is the radius)}`

`\textsf{Area of a triangle}=\sf \frac12bh \quad \textsf{(where b is the base and h is the height)}`

Given:

• 
  `\pi` = 3.14
• r = 6 cm
• b = 12 cm
• h = 6 cm

`\begin{aligned}\implies \textsf{Shaded area} & =\sf \frac12\pi r^2-\sf \frac12bh\\ & = \sf \frac12 \cdot 3.14 \cdot 6^2-\frac12 \cdot 12 \cdot 6\\ & = \sf 56.52-36\\ ^& = \sf 20.52\:\:cm^2\end{aligned}`