Question

A rectangle foot ball fields is 64 yards wide and 100 yards long. A player runs from one corner of the field in a diagonal line to the opposite corner. How far did the player run? Round your answer to the nearest yard.

Answer 1

The player ran 119 yards

Explanation:

The diagram below represents the problem.

The rectangular football fields is 64 yards wide and 100 yards long. A player ran the length of the diagonal.

Hence, we need to find the length of the diagonal of the rectangle.

The width, length and diagonal of the football field form a right-angled triangle, with opposite as 64 yards, adjacent as 100 yards and hypotenuse as x.

Applying Pythagoras' theorem, we have that:

`hyp^2 = opp^2 + adj^2`

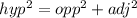
Therefore, the distance the player ran is:

`x^2 = 64^2 + 100^2\\\\x^2 = 4096 + 10000\\\\x^2 = 14096\\\\x = √(14096) \\\\x= 119 yards`

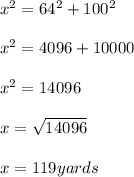
The player ran 119 yards.