Question

A flagpole broke in a storm. It was originally 81 8181 feet tall. 28 2828 feet are still sticking straight out of the ground, where it snapped, but the remaining piece has hinged over and touches the ground some distance away. How far away is the end of the pole from the base of the pole along the ground?

Answer 1

45 feet

Explanation:

Hey there!

In order to find out the answer, we need to first picture the triangle in our heads. Then we use the Pythagorean theorem to find out the missing side.

So with the given information, we can tell that one of the legs of the triangle is 28 feet tall and the hypotenuse is 53 feet long

Now we can input this into the Pythagorean formula:

`A^2+B^2=C^2 \\ 28^2 + B^2 = 53^2 \\ 784 + B^2 = 2809 \\ B^2 = 2809-784 \\ B^2 = 2025 \\ √(B^2)=√(2025) \\ B = 45`

So, the pole is 45 feet away from the base of the pole

Answer 2

The end of the flagpole is 50.79 ft away from the base of the pole.

Explanation:

The problem is represented by the diagram below.

The broken flagpole forms the shape of a right angled triangle. We need to find one of the sides of the triangle, the adjacent (x).

The hypotenuse is the broken part of the flagpole (53 ft), while the opposite is the part of the flagpole that is still stuck to the ground (28 ft).

Using Pythagoras theorem, we have that:

`hyp^2 = adj^2 + opp^2`

=>
`53^2 = x^2 + 28^2`

`3364 = x^2 + 784\\\\=> x^2 = 3364 - 784\\\\x^2 = 2580\\\\x = √(2580)\\ \\x = 50.79 ft`

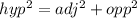
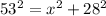
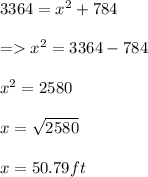
The end of the flagpole is 50.79 ft away from the base of the pole.