Answer:
B-The graph is incorrect. He should have shaded to the right of –8.
Explanation:
We examine the steps Alfonso follows in graphing the inequality x > -8.
Step 1: Draw a number line, and place an open circle at –8.
A number line going from negative 13 to negative 3. An open circle is at negative 8.
The first step is correct, since we use an open circle for > or < and a closed circle for
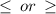 .
.
Step 2: Shade to the left of –8 to represent the infinite solutions to x greater-than negative 8.
This step is incorrect since values to the left are always less than.
He should have shaded to the right of -8.
The error made is Option B.
CHECK:
x=-7
-7>-8 (TRUE)