Answer:
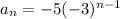
Explanation:
A geometric sequence or progession is defined as a sequence where each term can be found by multiplying a factor with the first term.
In this case, the first term is -5, the second term is 15 and the third term is -45.
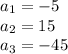
To find the factor, we divide the second term by the first, and the third term by the second.
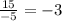
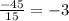
As you can notice, the factor is -3, so
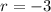 .
.
Now, the explicit formula of this sequence can be found with
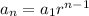
Where
 refers to the n-th term.
refers to the n-th term.
Replacing values, we have
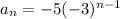
Therefore, the explicit formula of the geometric sequence is
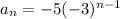
Which can be used to find any other term in the sequence.