Question

Find the exact length of the third side

Answer 1

8

Explanation:

This is a right triangle, and we know this because of the little square in the corner. Therefore, we can use the Pythagorean theorem.

a^2+b^2=c^2

a and b are the legs, and c is the hypotenuse.

We know the 6 and the unknown side are the legs, since they form the right angle. We also know that 10 is the hypotenuse, since it is opposite the right angle.

6^2+b^2=10^2

Evaluate the exponents

36+b^2=100

Now, we need to solve for b. To do this, we need to get by itself. First, subtract 36 from both sides

36-36+b^2=100-36

b^2=64

Take the square root of both sides

`√(b^2)=√(64)`

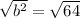
b=8

So, the the unknown side is 8

Answer 2

X is 8

Explanation:

`100^(2) =6^(2) +x^(2)`

`x=8`