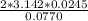Answer:
The phase difference is

Step-by-step explanation:
From the question we are told that
The distance between the loudspeakers is
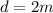
The distance of the listener from the wall

The frequency of the loudspeakers is
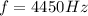
The velocity of sound is
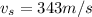
The path difference of the sound wave that is getting to the listener is mathematically represented as
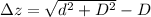
Substituting values
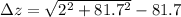
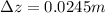
The phase difference is mathematically represented as
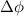 =
=
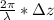
Where
 is the wavelength which is mathematically represented as
is the wavelength which is mathematically represented as
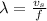
substituting value

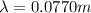
Substituting value into the equation for phase difference
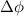 =
=