Solve the system of equations: y= 2x - 2 y= x2 - x-6 O A. (-1,-5) and (4,2) O B. (0, -2) and (2, 2) O C. (-1,-4) and (4, 6) D. (-2,0) and (3,0)

Question

asked Jan 10, 2023 233k views

1 Answer

Gletscher · Answer 1 · 2023-01-14T11:31:37+0000

Answer:

C) (-1, -4) and (4, 6)

Explanation:

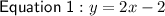
$\textsf{Equation 1}:y=2x-2$

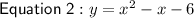
$\textsf{Equation 2}:y=x^2-x-6$

Substitute Equation 1 into Equation 2 and solve for x:

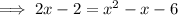
$\implies 2x-2=x^2-x-6$

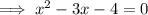
$\implies x^2-3x-4=0$

Find two numbers that multiply to -4 and sum to -3: -4 and 1

Rewrite the middle term as the sum of these two numbers:

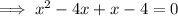
$\implies x^2-4x+x-4=0$

Factorize the first two terms and the last two terms separately:

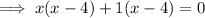
$\implies x(x-4)+1(x-4)=0$

Factor out the common term
(x-4) :

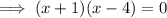
$\implies (x+1)(x-4)=0$

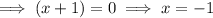
$\implies (x+1)=0 \implies x=-1$

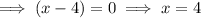
$\implies (x-4)=0 \implies x=4$

Substitute the found values of x into Equation 1 and solve for y:

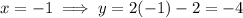
$x=-1 \implies y=2(-1)-2=-4$

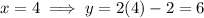
$x=4 \implies y=2(4)-2=6$

Therefore, the solution to the system of equations is:

(-1, -4) and (4, 6)