Answer:
The volume of cone is 476.61 cm³.
Step-by-step explanation:
Solution :
As per given question we have provided :
 Slant height = 14 cm
Slant height = 14 cm
 Radius = 6 cm
Radius = 6 cm

Firstly, finding the height of cone by substituting the values in the formula :
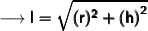
 Slant height (l) = 14 cm
Slant height (l) = 14 cm
 Radius (r) = 6 cm
Radius (r) = 6 cm
 Height (h) = ?
Height (h) = ?
Substituting all the given values in the formula to find the height of cone :
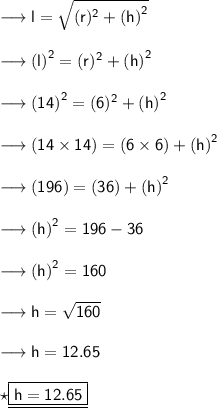
Hence, the height of cone is 12.65 cm.

Now, finding the volume of cone by substituting the values in the formula :
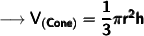
 V = Volume
V = Volume
 π = 3.14
π = 3.14
 r = radius
r = radius
 h = height
h = height
Substituting all the given values in the formula to find the volume of cone :

Hence, the volume of cone is 476.61 cm³.
