Answer:
The ratio of the surface area of the smaller sphere to the surface area of the larger sphere is 25:64.
Explanation:
The surface area of square is given by formula as follows :
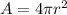
r is radius of sphere
The ratio of the lengths of the radii of two spheres is 5 : 8. The ratio of the surface area of the smaller sphere to the surface area of the larger sphere is :

Here,
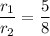
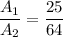
So, the ratio of the surface area of the smaller sphere to the surface area of the larger sphere is 25:64.