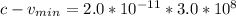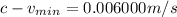Question

Earth's neighboring galaxy, the Andromeda Galaxy, is a distance of 2.54×1072.54×107 light-years from Earth. If the lifetime of a human is taken to be 75.075.0 years, a spaceship would need to achieve some minimum speed vminvmin to deliver a living human being to this galaxy. How close to the speed of light would this minimum speed be? Express your answer as the difference between vminvmin and the speed of light cc .

Answer 1

The closeness to the speed of light is
`c -v_(min) =0.006000m/s`

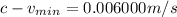
Step-by-step explanation:

From the question we are told that

The time taken to travel to Andromeda Galaxy is
`t_o = 2.54* 10^7 \ light -years`

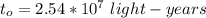
The life time of human is
`t = 75 \ years`

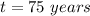
Generally the life time of a human can evaluated mathematically as follows

`t = t_o \sqrt{1 - (v_(min))/(c^2) }`

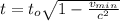
Where t is the life time of human

Making
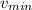`v_(min)` the subject of the formula

`v_(min) = [ \sqrt{1- ((t)/(t_o))^2 } ] c`

substituting values

`v_(min) = [ \sqrt {1- {((75.0)/(2.54*10^(7) ))^2 } ]} c`

`v_(min) = [ \sqrt{ 1 - 8.7202 *10^(-12)} ] c`

`v_(min) = 0.99999999998 c`

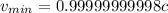
The closeness of
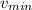`v_(min)` to the speed of light is mathematically evaluated as

`c -v_(min) = c - 0.99999999998c`

`c -v_(min) =( 1 - 0.99999999998) c`

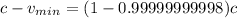
substituting
`3.0*10^(8)m/s` for c

`c -v_(min) =2.0*10^(-11) * 3.0*10^8`

`c -v_(min) =0.006000m/s`