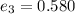Answer:
The emissivity of the radiation shield is
Step-by-step explanation:
From the question we are told that
The temperature of the first parallel plate is
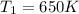
The temperature of the second parallel plate is
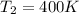
The emissivity of first plate is

The emissivity of first plate is

Generally the total radiation heat that is been transferred without the shield is mathematically represented as
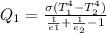
Where
 is the Stefan-Boltzmann constant which has a value
is the Stefan-Boltzmann constant which has a value
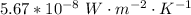
Substituting values
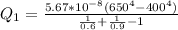
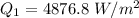
From the question we are told the that using the radiation shield would reduce the radiation heat transfer by 15%
So the new heat transfer is
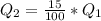
So
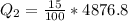
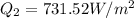
Now this new radiation heat transfer can be mathematically represented as
![Q_2 = (\sigma (T_1 ^4 - T_2 ^4))/( [(1)/(e_1 ) + (1)/(e_2 ) - 1 ] + n [(1)/(e_3) + (1)/(e_3) -1 ])](https://img.qammunity.org/2021/formulas/physics/college/h3697iv9d2l9gmrk4e4u09oe478gjvwd6h.png)
Where
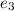 the emissivity of the radiation shield and n is the number of radiation shield
the emissivity of the radiation shield and n is the number of radiation shield
Substituting values
![731.52 = (5.67 *10^(-8) (650 ^4 - 400 ^4))/( [(1)/(0.6) + (1)/(0.9 ) - 1 ] + 1 [(1)/(e_3) + (1)/(e_3) -1 ])](https://img.qammunity.org/2021/formulas/physics/college/bawyu70et2jr2mgwoirtjusaxthkbax1jk.png)
![731.52 = (1.4175*10^(-5))/( [(1)/(0.6) + (1)/(0.9 ) - 1 ] + 1 [(1)/(e_3) + (1)/(e_3) -1 ])](https://img.qammunity.org/2021/formulas/physics/college/ngywl93hddlrmqijom8924cs8h4svl3hod.png)
![[(1)/(0.6) + (1)/(0.9 ) - 1 ] + 1 [(1)/(e_3) + (1)/(e_3) -1 ] = (1.4175*10^(-5))/(731.52)](https://img.qammunity.org/2021/formulas/physics/college/rdrgx66g85g53t9z8zxft4vz9oozkp4tma.png)
![[(1)/(0.6) + (1)/(0.9 ) - 1 ] + 1 [(1)/(e_3) + (1)/(e_3) -1 ] = 1.9377*10^(-8)](https://img.qammunity.org/2021/formulas/physics/college/dllmlvyaqqin3cpfw1wrd1fvsvasi44205.png)
![1 [(1)/(e_3) + (1)/(e_3) -1 ] = 1.9377*10^(-8) - [(1)/(0.6) + (1)/(0.9 ) - 1 ]](https://img.qammunity.org/2021/formulas/physics/college/dvb9o24qmb9g9klm77ma9fcg25yt4pgip8.png)