Question

To construct a confidence interval using the given confidence​ level, do whichever of the following is appropriate.​ (a) Find the critical value z Subscript alpha divided by 2​, ​(b) find the critical value t Subscript alpha divided by 2​, or​ (c) state that neither the normal nor the t distribution applies. 95​%; nequals200​; sigma equals 19.0​; population appears to be skewe

Answer 1

The correct option is (a).

Explanation:

According to the Central Limit Theorem if we have a population with mean μ and standard deviation σ and take appropriately huge random-samples (n > 30) from the population with replacement, then the distribution of the sample- means will be approximately normally-distributed.

Then, the mean of the sample means is given by,

`\mu_(\bar x)=\mu`

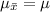
And the standard deviation of the sample means is given by,

`\sigma_(\bar x)=(\sigma)/(√(n))`

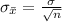
The information provided is:

n = 200

σ = 19.0

Population is skewed.

As the sample selected is quite large, i.e. n = 200 > 30 the central limit theorem can be used to approximate the distribution of the sample mean by the normal distribution.

So,
`\bar X\sim N(\mu, (\sigma^(2))/(n))`.

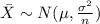Then to construct a confidence interval for mean we will use a z-interval.

And for 95% confidence level we will compute the critical value of z, i.e.
`z_(\alpha/2)`.

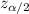Thus, the correct option is (a).