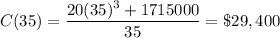Question

A closed box with a square base is to have a volume of 171 comma 500 cm cubed. The material for the top and bottom of the box costs ​$10.00 per square​ centimeter, while the material for the sides costs ​$2.50 per square centimeter. Find the dimensions of the box that will lead to the minimum total cost. What is the minimum total​ cost? Write an equation for​ C(x), the cost of the box as a function of​ x, the length of a side of the base. ​C(x)equals nothing

Answer 1

`C(x)=(20x^3+1715000)/(x)\\$Minimum cost, C(35)=\$29,400`

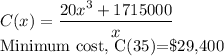
The dimensions that will lead to minimum cost of the box are a base length of 35 cm and a height of 140 cm.

Explanation:

Volume of the Square-Based box=171,500 cubic cm

Let the length of a side of the base=x cm

Volume
`=x^2h`

`x^2h=171,500\\h=(171500)/(x^2)`

The material for the top and bottom of the box costs ​$10.00 per square​ centimeter.

Surface Area of the Top and Bottom
`=2x^2`

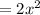
Therefore, Cost of the Top and Bottom
`=\$10X2x^2=20x^2`

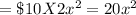
The material for the sides costs ​$2.50 per square centimeter.

Surface Area of the Sides=4xh

Cost of the sides=$2.50 X 4xh =10xh

`\text{Substitute h}$=(171500)/(x^2) $into 10xh\\Cost of the sides=10x((171500)/(x^2))=(1715000)/(x)`

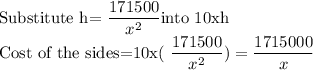
Therefore, total Cost of the box

`= 20x^2+(1715000)/(x)\\C(x)=(20x^3+1715000)/(x)`

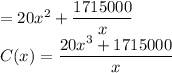
To find the minimum total cost, we solve for the critical points of C(x). This is obtained by equating its derivative to zero and solving for x.

`C'(x)=(40x^3-1715000)/(x^2)\\(40x^3-1715000)/(x^2)=0\\40x^3-1715000=0\\40x^3=1715000\\x^3=1715000/ 40\\x^3=42875\\x=\sqrt[3]{42875}=35`

Recall that:

`h=(171500)/(x^2)\\Therefore:\\h=(171500)/(35^2)=140cm`

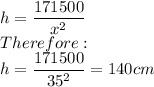
The dimensions that will lead to minimum costs are base length of 35cm and height of 140cm.

Therefore, the minimum total cost, at x=35cm

`C(35)=(20(35)^3+1715000)/(35)=\$29,400`