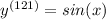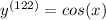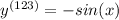Question

For the equation y = sin(x). Determine
`y^((123))` (the 123rd derivative of y)

Answer 1

-cos(x)

Explanation:

y¹ = cos(x)

y² = -sin(x)

y³ = -cos(x)

y⁴ = sin(x)

Every 4th derivative is sin(x)

y¹²⁰ = sin(x)

y¹²¹ = cos(x)

y¹²² = -sin(x)

y¹²³ = -cos(x)

Answer 2

-sin(x)

Explanation:

We have y = sin(x). The derivative of sin is cos, so:

y' = cos(x)

The derivative of cos is -sin, so:

y" = -sin(x)

Keep doing this, and we'll find a pattern:

y"' = -cos(x)

y"" = sin(x)

Now, we see the pattern; this is a cycle that repeats every 4:

sin(x), cos(x), -sin(x), -cos(x), sin(x), cos(x), -sin(x), -cos(x), ...

That means the derivative of y that is a multiple of 4 will always be equal to -cos(x). 120 is a multiple of 4, which means
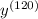`y^((120))` = -cos(x). Then:

`y^((121))=sin(x)`

`y^((122))=cos(x)`

`y^((123))=-sin(x)`