Answer:
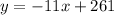
Explanation:
As you can observe in the image attached, the line that best fits passes through point B and C. That means we can use those point to find the slope of such line.

Where
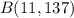 and
and
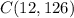
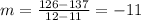
So, the slope of the line that best fits is -11, approximately.
Now, we use the point-slope formula to find the equation.
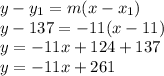
Therefore, the line that best fits is
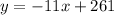 approximately.
approximately.
Remember, when we estimate a line for some data on a scatterplot, we are calculating an approximation, that's why we also said "approximately", because the line is an approximation where the majority of point meet.