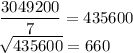By what least number should 3049200 be divided to get a perfect square? Please help me with this question Thanks in advance

Question

asked Sep 22, 2021 164k views

1 Answer

Havaligi Sathish · Answer 1 · 2021-09-27T15:01:42+0000

Answer:

7

Explanation:

Given the number: 3049200

To determine the least number by which we must divide to obtain a perfect square, we first express the number as a product of its prime factors.

3049200=2 x 2 x 2 x 2 x 3 x 3 x 5 x 5 x 7 x 11 x 11

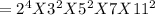
=2^4 X 3^2 X 5^2 X 7 X 11^2

If you observe the product in exponent form, only the power of 7 is odd. Every other number is a perfect square.

Therefore, if we divide by 7, the result is a perfect square.

CHECK

$(3049200)/(7) =435600\\√(435600)=660$