Answer:
(a) 10.20 s (nearest hundredth)
(b) 127.55 m (nearest hundredth)
(c) 883.70 m (nearest hundredth)
Step-by-step explanation:
Part (a)
At the end of the projectile's flight, its vertical displacement will be zero.
Resolving vertically, taking up as positive:
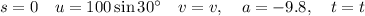
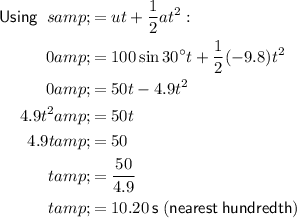
Part (b)
At the maximum height, vertical velocity will be zero.
Resolving vertically, taking up as positive:
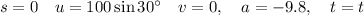

Part (c)
The horizontal velocity of a projectile is always constant, so u = v.
The horizontal component of acceleration is zero.
Resolving horizontally, taking right as positive (and using the value for t we found in part a):

