Answer:
the correct answer is: more than 5 meters of particular m1, but less than 10 m of the particle m₂
Step-by-step explanation:
The center of mass, the point of a system where all external forces can be applied, is defined by
 = 1 /M ∑
= 1 /M ∑
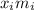
where M is the total mass of the system, x_{i} m_{i} are the position and mass of each item in the system,
let's apply this equation to our case
the total mass is
M = m₁ + m₂
for the calculations we must fix a reference system, we will place it in the second mass (m₂)
x_{cm}= 1/M (m₁ d + m₂ 0)
where d is the distance between the two masses in this case d = 10 m
x_{cm} = m₁ / (m₁ + m₂) d
x_{cm} = m₁ / (m₁ + m₂) 10
as the mass m₁ <m₂
Let us analyze the answer if the masses sides were equal the center of mass would be x_{cm} = 5 m, but since m₁ <m₂ the center of mass must be closer to m₂.
Therefore the correct answer is: more than 5 meters of particular m1, but less than 10 m of the particle m₂