Answer:

And olving for the deviation we got:
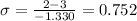
And then we want to find this probability:
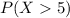
Using the z score we got:
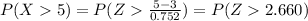
And using the complement rule and the normal standard distirbution or excel we got:
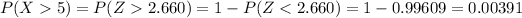
And the best answer for this case is:
(A) 0.0039
Explanation:
For this case we define the random variable X as " The nitrite level" and we know that the distribution of X is given by:
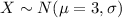
We don't know the deviation. We also know the following condition:
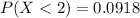
So then we can use the z score formula given by:
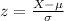
We need to find a z score value that accumulates 0.0918 of the area on the right and we got z = -1.330, since P(Z<-1.330) = 0.0918. Then we can set up the following equation:

And olving for the deviation we got:
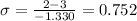
And then we want to find this probability:
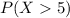
Using the z score we got:
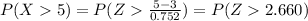
And using the complement rule and the normal standard distirbution or excel we got:
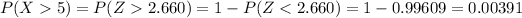
And the best answer for this case is:
(A) 0.0039