Answer:
B
Explanation:
Currently, the graph looks like the attachment.
The centre of this circle is at (-4, -2), but when we flip it over the line x = 1 (the blue line on the picture), the centre will become ((1- -4) + 1, -2) = (6, -2).
Look for the equation
 that matches this center. We want the center (h, k) to be (6, -2), so our equation should look something like:
that matches this center. We want the center (h, k) to be (6, -2), so our equation should look something like:
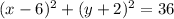
The answer is B.